 |
 |
|
|
Optics of Progressive LensesBy Darryl Meister IntroductionThis course will present the fundamental principles of progressive lens optics and design. This is a technical, advanced level course intended for dispensing opticians, laboratory technicians, and paraoptometric personnel. An understanding of both basic algebra and intermediate optical theory is required. BackgroundAlthough progressive addition lenses (or "PALs") didn't enjoy commercial success until the 1960s, the concept has been around for nearly a century. Owen Aves, co-founder of London's Institute of Optometry, patented a progressive lens design in 1907. Because of the dual-surface nature of this lens design, it was limited to spherical prescriptions. Shortly after, Henry Gowlland patented improved progressive lens designs in 1909 and 1914, which utilized a conicoidal back surface to produce the addition power. Estelle Glancy at American Optical patented one of the earliest progressive lens designs that relied exclusively on a single progressive front surface in 1923. However, early lens designs generally proved impractical to produce in prescription form in mass quantity with the technology available at the time. The first commercially successful progressive lens in Europe, Essel's Varilux lens, was introduced in 1959. The Univis Omnifocal, introduced in 1965, became the first commercially successful lens in the United States. The success of these lenses was due in no small part to technical achievements in fabricating asymmetric surfaces on a large-scale production basis, often relying on clever grinding assemblies. Since the 1960s, progressive lens usage has increased rapidly as the designs continued to improve. Today, over half of all multifocals sold are progressive lenses. 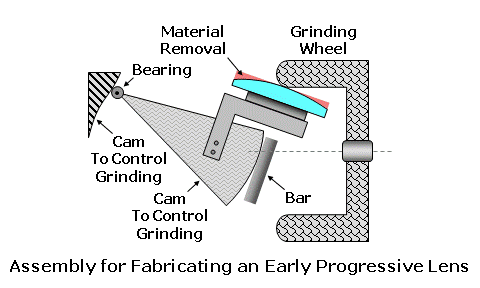 Arguably, well-designed progressive lenses replicate natural vision more effectively than traditional (lined) bifocals, since they provide smooth changes in vision and a full range of focus. In fact, according to some clinical studies, progressive lenses actually have a higher rate of adaptation than traditional bifocals, and are preferred to traditional bifocals 4-to-1 by wearers. We can summarize the primary benefits of progressive lenses as follows:
 This course will focus on the optics of traditional, general-purpose progressive lens designs. However, the principles discussed here apply to virtually all classes of progressive lenses. In addition to traditional progressive lenses, there are several "specialty" classes of progressives that are worth mentioning:
The Progressive ProblemMost opticians are (painfully) aware of the geometrical aspects of Executive- and Franklin-style bifocals, lenses producing both distance and reading power across the entire width of the surface. The surface of an Executive-style bifocal is essentially a section from two conjoined hemispheres of differing surface curvature. A flatter distance curve, representing the major portion for the distance prescription, meets a steeper near curve, representing the segment for the Add power. The junction between these curves results in a prominent ledge across the surface. At the center of the lens, the two front curves of the front surface meet at only a single point, and are only contiguous (or unbroken) at that point. Away from this point, the individual curves gradually break farther and farther apart as the near curve steepens more quickly than the distance curve, resulting in the infamous edge profile typical of Executive-style bifocals. This ledge represents an increasing discontinuity as the surface breaks farther and farther apart.  However, we can reduce this ledge by inserting an intermediate curve between the distance and near curves, with a surface curvature that is slightly steeper than the distance curve. The addition of this intermediate curve converts our bifocal into an Executive-style trifocal, complete with intermediate power for mid-range vision. Although this results in two ledges, each individual ledge is smaller is smaller than the original ledge. We can continue inserting narrow horizontal sections of spheres that represent additional intermediate curves, each progressively steeper than the last. If we do this an "N" number of times, we create an Executive-style N-focal. As with the bifocal (N = 2) and trifocal (N = 3), each subsequent spherical section of this N-focal is still only contiguous with the section above it at a single point. Moreover, a vertical meridian at the center of the lens surface is formed that contains the locus of these contiguous points. This vertical meridian is known as the umbilic of the lens surface. 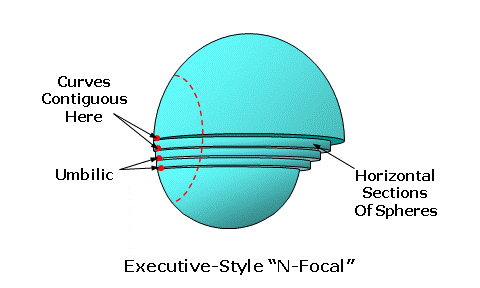 The addition of more and more, narrower and narrower horizontal sections continues to reduce the individual heights of the ledges separating these curves. As the number of these horizontal sections increases to infinity, the individual ledge heights approach zero. Further, once these heights reach zero, the final surface becomes perfectly smooth and continuous, with no visible junctions between the curves. We have now created a very simple progressive lens surface compromising a distance zone, a near zone, and a progressive section connecting the two. The curvature of this very basic progressive surface increases smoothly from the distance to the near regions, providing a gradual change in power as well as mid-range vision. You can verify this gradual change in surface power with a common lens clock (or lens measure). The vertical curvature will vary from its lowest (flattest) value in the distance zone to its highest (steepest) value in the near zone; the difference between the two will be equal to the Add power of the surface. Moreover, the horizontal curvature of this surface is equal to its vertical curvature at any single, infinitely small point. 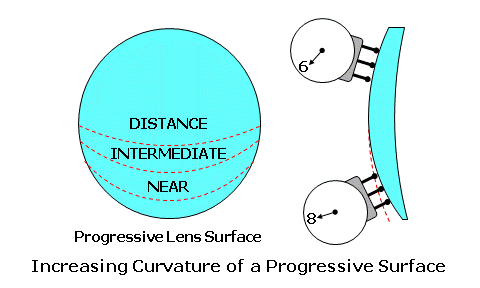 Unfortunately, while the horizontal curvature of this surface is equal to its vertical curvature at any point, the curvatures in oblique meridians at these points are not equal in the peripheral regions of the progressive section. In fact, each point actually behaves like a tiny cylinder lens oriented near either axis 45° or axis 135°. These oblique curvatures are only completely equal to each other along the single, vertical umbilic meridian of the lens. Away from the umbilic meridian, in the lateral regions of the lens where the ledges of our infinite N-Focal have essentially been "blended" together, the minimum and maximum surface curvatures grow farther and farther apart. In ophthalmic optics, a surface that produces both a minimum curvature and a maximum curvature at the same point is referred to as a toric surface. The difference in surface curvatures on a toric surface results in cylinder power, which in turn produces an astigmatic focus. This situation is analogous to spectacle lenses, which employ a toric surface in order to produce cylinder power. Consequently, we can say that points in the "blending" regions of a progressive lens surface are locally toric. However, we more commonly refer to this difference in surface curvature as surface astigmatism, since the surface produces an astigmatic focus at these points. In summary, while the curvature in the horizontal and vertical meridians at any point on the simple progressive lens surface described here depends only on the vertical location on the lens surface, the minimum and maximum curvatures of the surface at any point—which occur in oblique meridians—also depend upon the horizontal location on the surface. Further, the curvatures at any point in the progressive blending region of the lens are only equal along the vertical umbilic meridian. Away from the umbilic meridian of the lens, the curvatures in oblique meridians (near axis 45° and axis 135°) immediately start to differ, and this difference increases toward the periphery of the lens. This results in the characteristic surface astigmatism inherent in progressive lenses. The basic progressive lens we have examined here, which employs circular cross-sections (that is, horizontal sections of spheres), suffers from a great deal of unwanted astigmatism in the lateral blending regions of the surface. The wearer will perceive the effects of this surface astigmatism as blur and distortion. Fortunately, use of more complex surfaces can reduce this surface astigmatism. For instance, replacing the circular cross-sections with conic sections, including ellipses, hyperpolas, and parabolas, will significantly reduce the levels of unwanted astigmatism. Indeed, one of the primary goals of progressive lens design is the minimization and sensible distribution of this unwanted surface astigmatism. Surface AstigmatismIn order to better understand the presence of surface astigmatism in progressive lenses and to develop a more solid intuition regarding the optics of progressive lenses, let us return to our Executive-style bifocal. How could we go about "blending" the two hemispheres together in order to produce a smooth, continuous surface? That is to say, how could we "fill in" the region beneath the ledge that exists between the flatter distance curve and the steeper near curve? We examined a progressive surface produced by making an "infinite" N-focal, but we will now consider a lens design that may be simpler to imagine. For simplicity, let us visualize an Executive-style bifocal with a Plano (flat) back curve and a Plano front curve in the distance region. In this case, the near region of the lens will have a front surface roughly equal to its Add Power, while the distance zone will be perfectly flat. We will now remove a 90° wedge from the side of this lens. The cross-section of the lens formed by this missing wedge is now similar to a plano plus-cylinder that has been cut in half. Note that the surface astigmatism of a plano cylinder is equal to the surface power of the cylinder across its meridian of curvature—or power meridian. Now visualize a plano plus-cylinder lens equal in power to the bifocal segment (or Add power). Such a plus-cylinder lens will be flat (Plano) and produce no power along its axis meridian, while it will produce its maximum plus power through its power meridian. We will take this plano plus-cylinder, cut it in half along its axis (flat) meridian, and then insert it into the space left by our missing wedge. It should be a perfect fit. Essentially, we are showing that it is possible to "blend" the flatter distance portion to the steeper bifocal portion with the use of cylinder power (at an oblique axis). 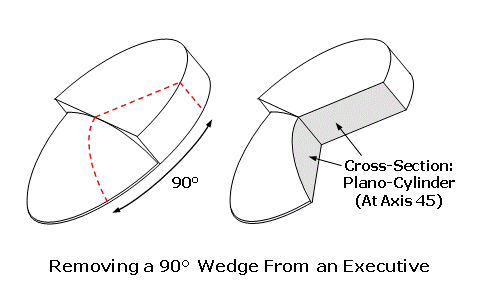 Finally, we can simply trim away the excess material. As previously noted, the cylinder power of this plano plus-cylinder will be equal to the power of the bifocal segment, since the power curve of this cylinder is in fact an extension of the curve of the segment. Moreover, it should be apparent that this cylinder has no power along the axis meridian (that is, it's a plano cylinder) because this meridian is an extension of the Plano distance curve. Further, this cylinder will be oriented at axis 45° (the angle the wedge makes in the distance). Of course, when we repeat this process for the other side of the lens, the cylinder will be oriented at axis 135°. 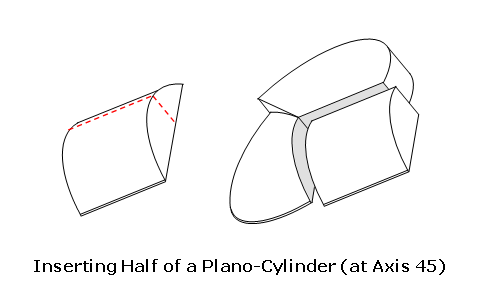 We have now created a "No-Line" Executive-style bifocal. While this isn't a true progressive lens, since it has no progressive change in surface curvature, it demonstrates geometrically how surface astigmatism can smoothly blend two hemispheres of differing curvature—the flatter distance curve and the steeper near curve. This lens design also provides some intuition about the surface astigmatism of progressive lenses, in general, since they both share the following characteristics:
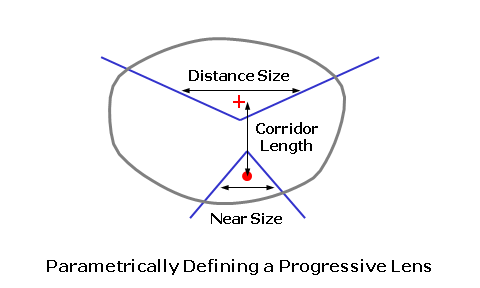
Anatomy of a Progressive LensThe proceeding sections will frequently reference various regions of progressive lenses, so a brief overview of the "gross anatomy" of a typical progressive lens surface is in order. General-purpose progressive lenses belong to a class of surfaces that offer four very important structural features:
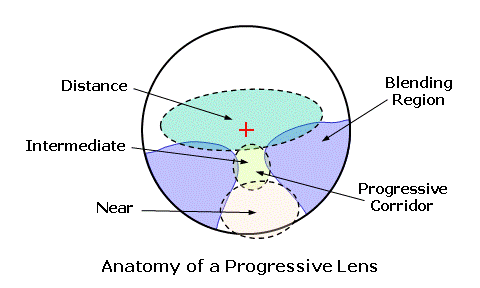 Progressive addition lenses are supplied with two types of marking for layout, power verification, dispensing, and identification purposes. Removable markings, which are ink markings stamped onto the lens, identify the layout, verification, and dispensing points of the lens. In addition, permanent markings, which are etched upon the lens surface, provide the brand identification and Add power of the lens, as well as alignment reference markings—which are 34 mm apart and used to reapply the ink markings when necessary. The removable ink markings indicate the locations of the cardinal reference points of the progressive lens design:
 Characterizing Progressive OpticsRecall that any arbitrary point on a progressive lens surface contains both a maximum and a minimum surface curvature, which are perpendicular to each other. Essentially, each point is locally toric, similar to a lens with cylinder power. The maximum and minimum surface curvatures at this point produce maximum and minimum surface powers, which we will refer to as the principal surface powers, just as the two planes containing the maximum and minimum powers in a cylinder lens are referred to as the principal meridians. 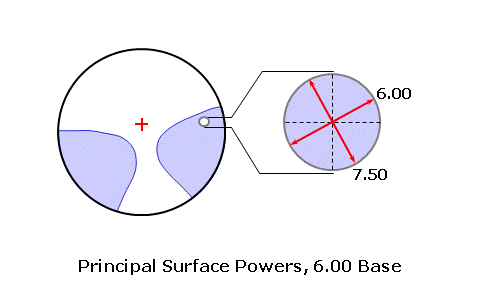 For example, consider a point on a progressive lens surface that produces a minimum principal power (P1) of +6.00 D and a maximum principal power (P2) of +7.50 D. The surface astigmatism at this point is equal to the difference between these two powers, or P2 - P1 = 7.50 - 6.00 = 1.50 D. The mean power of the surface at this point is equal to the average of these two powers, or ½ (P1 + P2) = ½ (6.00 + 7.50) = +6.75 D. Astigmatism is comparable to cylinder power, while mean power is comparable to sphere power. Traditionally, the mean power is offset by the value of the Base curve—or offset by the power at the distance reference point—in order to arrive at the net plus power produced by the design once the lens has been surfaced to the desired prescription. This produces a more meaningful distribution, since it shows the change in actual Add power of the progressive surface as experienced by the wearer. If the base curve is +6.00 D in our example, the mean power can be described as 6.75 - 6.00 = +0.75 D at this particular point. Contour plots are a type of graphical representation of a quantity or optical property that varies with two other variables, such as the vertical and horizontal coordinates of a lens surface. Contour plots are usually created by sampling a quantity over an array of regularly distributed points across the lens. Points of equal value for the quantity are then connected by curves, called contour lines. Generally, only contour lines at regularly spaced intervals are shown (e.g., 0.50, 1.00, 1.50, and so on), and the contour levels may also employ gradations of color. Most commonly, these plots are used to show the distribution of optical properties, particularly surface astigmatism and mean power, across the lens surface.  Astigmatism plots generally indicate regions of blur and image distortion, and can also give some indication as to the size of the distance, intermediate, and near zones. The spacing of the contours in these plots will also indicate how rapidly the unwanted astigmatism is increasing. Mean power plots can be used to determine the location of the full add power, as well as regions of excess plus power that may also result in blur—including unwanted plus power in the distance zone. It should be noted that, while plots of surface astigmatism and mean power are indicative of performance, they are not necessarily representative of performance. They provide a look at certain convenient physical quantities, but accurate interpretation of these plots requires some skill and experience. There are also other quantities of interest, including measures of binocular performance, image swim, and blur. Furthermore, plots that depict ray-traced quantities, which are calculated using lens-eye modeling of optical performance, generally provide more visually meaningful comparisons than plots of surface quantities. It is also possible to characterize the optics of a progressive lens surface using wavefront analysis, which considers higher-order aberrations—such as spherical aberration, coma, and trefoil—in addition to mean power and astigmatism. One particularly convenient way of expressing wavefront aberrations is with the use of a Zernike series, which is a series of polynomials whose terms represent quantities such as astigmatism, mean power error, forms of coma, and so on. The Progressive UmbilicRecall that a progressive lens surface contains a single vertical meridian that is free from surface astigmatism, known as the umbilic. Since the umbilic is free from surface astigmatism, the surface is locally spherical at each point along this meridian. Specifically, any point along the umbilic can be represented with an osculating (or "kissing") sphere equal in curvature to the curvature of the actual surface at that point, and tangent to the surface. The umbilic represents the centerline of the progressive corridor—or the channel of relatively clear vision connecting the distance and near viewing zones. The curvature down the umbilic steadily increases in order to produce a progressive increase in mean power from a point producing the lowest mean power (in the distance zone) to a point producing the highest mean power (in the near zone). The vertical distance between these two points is referred to as the corridor length of the lens. The total change in mean power along the umbilic between these two locations represents the specified Add power. While the horizontal, vertical, and oblique curvatures at any infinitely small point along the umbilic are equal, these curvatures start to differ away from the umbilic. The surface astigmatism, which is equal to the maximum difference between these curvatures at any point, rapidly increases as you move into the lateral blending regions of the lens. In the vicinity of the umbilic, this surface astigmatism actually increases at a very predictable rate away from the umbilic. Minkwitz showed that the rate of change in surface astigmatism (A) away from the umbilic grows at twice the rate of change in mean power (M) along the umbilic. Mathematically, this can be described as: ΔA/Δx ≈ 2 × ΔM/Δy Where (ΔA) is the change in astigmatism, (Δx) is the lateral distance away from the umbilic, (ΔM) is the change in mean power, and (Δy) is the vertical distance along the umbilic. If the horizontal distance (Δx) to a point (A) is equal to the vertical distance (Δy) to a point (M), we can assume that the change in surface astigmatism at point (A) is approximately equal to twice the change in mean power at point (M). 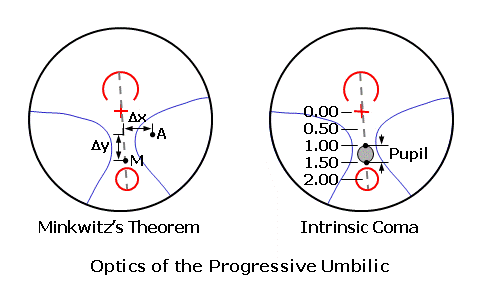 It is interesting to note that, although any infinitely small point along the umbilic is locally spherical, the curvatures immediately above and below this point will differ since the mean power of the surface is gradually changing along the umbilic. The pupil of the eye is 2 to 5 mm in diameter under typical lighting conditions, which means that eye samples an area of this size at any point on the surface—including points along the umbilic. Consequently, the refractive power of the surface will differ between the upper and lower margins of the pupil. This variation in refractive power across the pupil results in an imaging defect (or aberration) known as intrinsic coma. For instance, consider a simple progressive lens that produces an Add power of +2.00 D at a distance of 16 mm below the distance zone. If the umbilic has a linear change in power from distance to near, the mean power of the surface changes roughly 2.00 ÷ 16 = 0.125 D per millimeter. For a 4 mm pupil size, the difference in mean power between the upper and lower edges of the pupil is equal to 0.125 × 4 = 0.50 D. This 0.50 D variation in refractive power across the pupil creates a vertically oriented patch of blur on the retina. (Note that it does not produce a total power error of 0.50 D; the blur from this aberration is equivalent to a much smaller power error.) Fortunately, the blur produced by the intrinsic coma inherent in a typical progressive lens is generally well within the depth of focus of the eye, so it is not an issue for the wearer. Minkwitz's theorem provides us with a few useful guidelines regarding the optics of a progressive lens in the vicinity of the umbilic (progressive corridor):
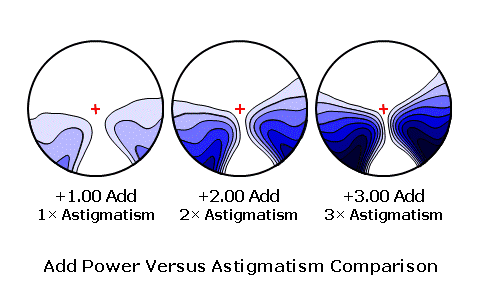 Distribution of Surface OpticsOne of the most fundamental aspects of the basic progressive lens design is the distribution of its surface optics, including power and astigmatism. These features define the gross performance of the lens, and represent a veritable "fingerprint" that distinguishes one progressive lens design from another. The magnitude, distribution, and gradient (or rate of change) of power and astigmatism are factors that affect the performance and wearer acceptance of the lens design. Progressive lenses are often arbitrarily classified into either one of two broad "philosophies" of lens design by the relative magnitude and distribution of their surface optics:
In general, harder progressive lens designs will provide wider fields of clear vision, at the expense of higher levels of swim, distortion, and blur. These lens designs will provide better central or foveal vision, which is important during tasks that involve critical viewing or that require good visual acuity. Additionally, harder lens designs frequently employ shorter, narrower progressive corridors. 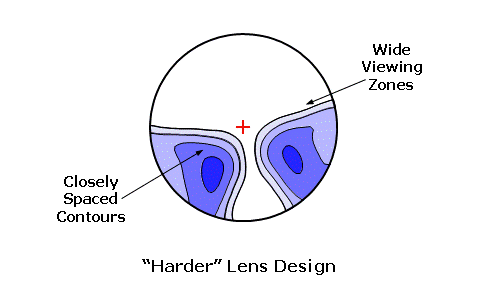 Softer lens designs, on the other hand, will provide reduced levels of astigmatism and swim, while limiting the size of the zones of clear vision. These lens designs will provide better peripheral or dynamic vision, which is important during tasks that involve active viewing. Softer designs tend to improve "comfort" and adaptation for emerging presbyopes, while harder designs offer more of the kind of utility current bifocal wearers enjoy. Softer lens designs often employ longer, wider progressive corridors as part of their overall design strategy. 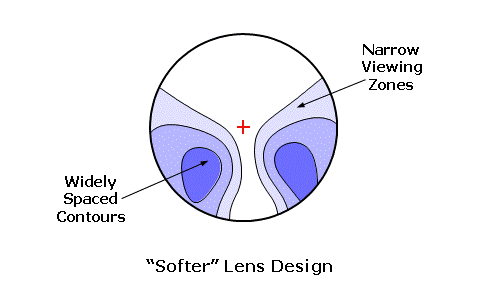 Essentially, as you increase the region of the lens used to "blend" the distance and near zones together—effectively spreading the blending region out over a larger area—you decrease the levels of surface astigmatism. Conversely, as you decrease the region of the lens used to blend these two zones, you increase the levels of surface astigmatism. Modern progressive lenses are seldom absolutely "hard" or absolutely "soft," but rather strive for a balance between the two in order to achieve better overall utility. A manufacturer may also choose to employ the features of a softer design in the distance periphery in order to improve dynamic peripheral vision, while employing the features of a harder design in the near periphery in order to ensure a wide field of near vision. This "hybrid"-like design is another approach that sensibly combines the best features of both philosophies. Progressive Lens DesignGeometrically, we often describe a progressive lens surface as a "locally toric" surface that is "globally smooth." A smooth surface must have a continuous 2nd derivative over the entire surface, which is related to the rate of change in the height (or sagitta) of the surface. A continuous 2nd derivative implies that the surface has a continous surface height and continuous 1st derivative, and ensures that the surface meets the following criteria:
Consequently, the primary goal of progressive lens design is to produce a smooth surface that possesses all the necessary structural features, including distance, intermediate, and near zones, with a minimum of aberrations, or optical imaging defects. Often, the first step in progressive lens design is to determine the performance requirements of the final lens design, including the length of the progressive corridor, the configuration of the central viewing zones, the distribution of optics in the periphery, and so on. Once these performance requirements are understood, they can be used to establish the various parameters that fundamentally define the basic progressive lens design:
 There are numerous approaches to defining mathematically a progressive lens surface. Depending upon the mathematical functions used by the lens designer to create the initial surface, the fundamental design parameters described above may be directly defined in the equations or arrived at experimentally by adjusting related parameters. If these functions are sophisticated enough to produce a sufficiently adequate and well-behaved progressive lens surface, the surface may be suitable for use without any significant refinements. However, this "starting" surface is commonly optimized—or optically refined—further in order to maximize performance or to achieve specific performance requirements. This is usually done numerically using lens design software that attempts to find a "real" surface that satisfies the ideal performance requirements of the lens design as closely as possible. Essentially, the software minimizes the differences between an actual smooth surface and a theoretical target surface. In many cases, this software employs a finite element method, which uses special functions to model surfaces and to find solutions to minimization problems. An initial starting surface is first specified and then modeled mathematically using the finite element method. The computational area of the lens surface is "discretized" by breaking regions of the surface up into square elements across a reference grid, or mesh. Each intersection between these square elements represents a position on the lens surface, and is referred to as a node. Each node contains an array of mathematical quantities that can characterize the surface at that point, including its local curvatures. These nodes are mathematically connected using basis functions known as splines. In regions on the surface where optical performance is more critical, or changing more rapidly, the mesh can be subdivided as needed into smaller square elements with additional nodes to allow tighter control of the surface in those regions. The surface characteristics at these nodes are smoothly connected by mathematical spline functions, which ensure that the surface maintains a continuous 2nd derivative between these points.  A target distribution of optical quantities, representing the ideal distribution of quantities such as mean power and astigmatism, is first determined for each node and its corresponding point on the lens surface. Generally, a smooth surface cannot achieve this target distribution—at least for every point. Finite element method seeks to minimize the difference between the desired optical performance at any point on the surface and the actual optical performance possible with a continuously smooth surface. This is accomplished by minimizing merit functions at each node, which are equations of the form: M = Σ Wi × (Ai - Ti)2 = W1 × (A1 - T1)2 + W2 × (A2 - T2)2 ... Where (M) is the value of the merit function to minimize at a given node location, (W) is the weighting—or importance factor—of the measurement, (A) is the actual value of the measurement, and (T) is the target value of the measurement at that point. Merit functions are used to find a "least squares" solution. Common measurement quantities to minimize may include mean power errors, astigmatism, gradients of power, and so on. The weightings for these quantities can vary as a function of the node location, allowing different regions of the lens surface to emphasize different performance attributes (e.g., blur in the central viewing zones and image swim in the periphery). The individual nodes, or even collections of nodes, can be weighted, as well. Some regions of the lens must maintain exact optical specifications. The central viewing zones, for instance, are more heavily weighted so that the analysis achieves the desired target performance in these regions—at the expense of the peripheral regions, if necessary. The goal of finite element method is to minimize the total sum of all merit functions across the entire mesh. The actual surface is repeatedly manipulated by the program in order to reduce the sum of the entire range of merit functions across all nodes. These iterations eventually result in a real surface that comes as close as possible to producing the target optical distribution. Essentially, the process seeks to find an actual progressive surface that provides the desired optics in the central viewing zones, while minimizing optical errors elsewhere as much as possible. The measurement quantities may be calculated directly from surface characteristics, such as the principal surface powers, or they may be derived after first ray tracing a lens with the intended surface characteristics. Ray tracing is the process of modeling the theoretical optical performance of the lens as perceived by the wearer with the lens in its intended position of wear—including vertex distance and pantoscopic tilt—by calculating the refraction of light rays passing through the lens from various object points. 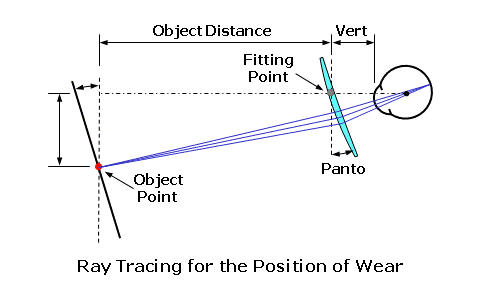 Once the lens design has been computed, a mold must be made. Surface description data are calculated for the lens design and then communicated to expensive milling machines. For metal molds, often used for injection-molded materials like polycarbonate, the mill may cut a progressive surface directly onto the metal mold. The mold is then polished to a high luster. For glass molds, often used for cast materials like hard resin, the mill may cut a progressive surface onto a ceramic former. A glass mold is then heated over the former until it softens and assumes the shape of the former in a process called slumping. Alternatively, the glass mold may be cut to the desired shape directly using a computer-controlled free-form or digital surfacing process. Once the mold has been made, some initial prototype lenses are produced. These lenses are then inspected to ensure that they achieve the desired optics. Often, because of lens material shrinkage and other variations in the manufacturing process, the initial lens design may need to be modified slightly in order to produce a manufactured lens blank that achieves the desired surface powers. Once the initial design has been "tweaked," a new mold is made and the inspection is repeated. This entire process must be performed for each Base curve and Add power combination, in every lens material. Since a typical progressive lens series may include up to 72 Base Curve and Add power combinations in up to 10 lens materials, this process requires a massive amount of development work and cost. The Progressive CorridorRecall that the umbilic represents the centerline of the progressive corridor, which is the channel of relatively clear vision connecting the distance and near viewing zones. It was also demonstrated mathematically that the width and intermediate utility of the progressive corridor depend upon the corridor length of the lens for a given Add power. The lens designer is primarily concerned with three features related to the geometry of the umbilic:
Earlier, we defined corridor length as the vertical distance between a point along the umbilic producing the lowest mean power (in the distance zone) to a point producing the highest mean power (in the near zone, where the maximum Add power occurs). The point producing the lowest surface power at the start of the umbilic is generally located at the distance reference point (DRP) of the lens, while the point producing the highest surface power at the end of the umbilic is generally located at the near reference point (NRP). In most cases, the umbilic is rotated so that it is aligned with the natural movement of the eyes as they converge to see nearby objects through the intermediate and near zones. This ensures that the eyepath, or the path the line of sight travels over the lens surface as it approaches the near zone, remains properly centered within the progressive corridor. As with lined multifocals, the lateral placement of the near zone is referred to as the inset. The total rotation of the umbilic will depend upon the inset. The eyepath of more advanced lens designs is precisely computed by calculating the theoretical reading distance that corresponds to the mean power at each point along the umbilic. The change in mean power (or Add power) along the umbilic is referred to as the power profile of the lens design. The power management geometry of the umbilic is primarily driven by the corridor length of the lens design, since the length of the corridor will dictate how rapidly the mean power must change in order to reach the full Add power. The average rate of change in mean power along the umbilic is inversely proportional to the corridor length: Rate of Change in Power = Add Power ÷ Corridor Length However, it is actually possible to vary the mean power along the umbilic in a non-linear fashion in order to achieve a degree of flexibility in the optical performance of the lens design. For instance, the power profile often ramps up in mean power rather slowly at first to minimize the excess plus power in the distance zone, which could otherwise blur vision. The power profile may then ramp up more quickly in the progressive corridor to deliver a sufficient amount of mean (Add) power for reading vision well above the near reference point, so that the wearer may realize some degree of near utility higher in the lens. Careful control of the power profile is also necessary to ensure that the change in Add power reflects the natural inclination of reading materials. 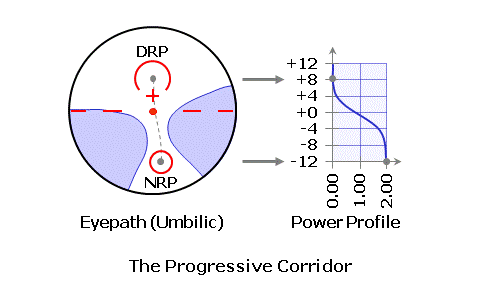 In practice, corridor length is measured from the fitting point, not the distance reference point, since the fitting point is aligned with the optical system of the eye. (The location of the distance reference point is somewhat arbitrary; it is generally located to ensure error-free verification of the prescription.) Furthermore, it is common to specify the length to a percentage of the specified Add power, such as 85% or 95%. Consequently, the advertised corridor length is often shorter than the distance to the near reference point. Although in theory a progressive lens could have a corridor length that approaches zero, there are certain optical and visual considerations that place practical limitations on the range of acceptable corridor lengths. Several factors must be taken into account when choosing the corridor length for a progressive lens design. For example, shorter corridor lengths offer the following advantages:
Every additional 1 mm of corridor length requires roughly 2° of additional ocular rotation to reach the near zone. If the corridor length is too long, the wearer may not be able to reach the full Add power of the near zone without awkward postural adjustments. However, shorter corridor lengths offer the following disadvantages:
The shorter the progressive corridor, the more the optics of the lens design must be effectively "squeezed" together. This results in higher levels of peripheral blur, reduced intermediate utility, or narrower viewing zones. Consequently, the length of the corridor should be carefully chosen in order to offer the most utility with the least amount of compromise. Viewing Zone ConfigurationUnlike lined multifocals, the central viewing zones of a progressive lens are not well-defined. The size and utility of the viewing zone will vary depending upon both the wearer's tolerance to blur and the nature of the visual task. For instance, the blur produced by unwanted astigmatism in the periphery of a progressive lens may make certain critical viewing tasks—such as reading—difficult, though these regions may be perfectly acceptable for dynamic vision, which often requires only localizing and recognizing objects. Nevertheless, the lens designer can define the size and shape of the central viewing zones by establishing a limit on the amount of acceptable power error, including the maximum levels of unwanted astigmatism and mean power error. (For our purposes, the mean power error represents the difference between the mean power produced by the lens design and the Add power actually required for a given reading distance.) For a given power error threshold, the size of the central viewing zones can be increased by pushing the unwanted astigmatism farther into the periphery. However, this trade-off results in a harder lens design with higher and more rapidly increasing levels of unwanted astigmatism. Because of this trade-off, lens designers must be careful not to increase the size of any particular central viewing zone any more than necessary. The size of each viewing zone should be judiciously managed in order to provide the best overall balance by maximizing the global utility of the lens design. Therefore, lens designers must choose the best balance between the size of the distance zone and the size of the near zone, so that both zones function comparably for the wearer. Of course, this will ultimately depend on the nature and frequency of the visual tasks performed by the wearer.  Some lens designers feel that maximized distance utility is preferred by wearers, and create progressive lenses with an emphasis on distance vision. These lens designs will have relatively large distance viewing zones, often at the expense of the near zone size. Other lens designers feel that maximized near utility is more useful to wearers, and create progressive lenses with an emphasis on near vision. Consequently, the idea of "balance" depends on how the lenses will be used by the actual wearer, not necessarily on the relative physical sizes of the viewing zones. Design of PeripheryUnfortunately, the surface astigmatism inherent in progressive lenses produces a significant amount of cylinder power, which the wearer perceives as blur. Clear vision through the peripheral regions of the lens, where this surface astigmatism is greatest, is often not possible because of this blur—particularly in higher Add powers. In addition to this blur, the changing prism and magnification produced by the power and astigmatism of a progressive lens can cause objects to appear to shift, distort, or even sway unnaturally in some cases. An annoying visual phenomenon may arise when the apparent movement of the visual environment through the lens differs from the physical movement detected by the neurophysical system because of variations in prism and magnification. The vestibular apparatus, which is located within the inner ear, is linked to the visual system and helps to both maintain your sense of balance and stabilize vision while in motion. The apparent "rocking" produced by this neurological conflict is known as image swim, and may result in a sensation of vertigo, or motion sickness. 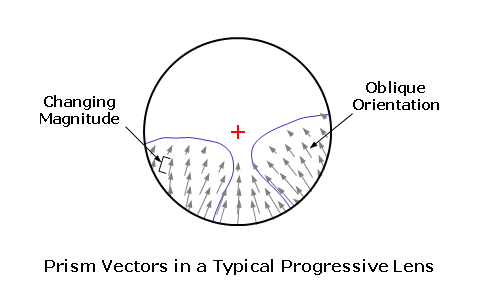 Objects—such as straight lines—may also appear curved or skewed when viewed through the lateral areas of the lens. This apparent shearing of images is called skew distortion, and occurs because the unwanted astigmatism in the periphery of progressive lenses causes unequal magnification in oblique directions. (Recall that the surface astigmatism of a progressive lens is generally obliquely oriented.) This unequal oblique magnification causes the vertical and horizontal lines of images to tilt and stretch (or skew). The more skewed a vertical line appears, the less orthoscopic—or straight—the image is through that particular zone. 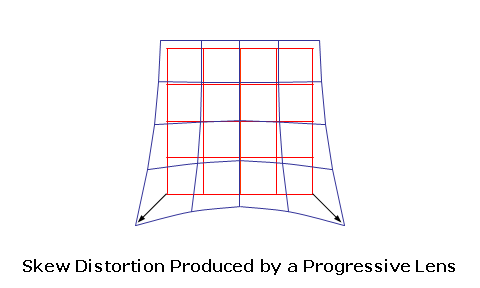 It should now be obvious that the design of the periphery of a progressive lens is critical to dynamic vision performance and wearer acceptance. Often, the distribution of optics in the periphery of a progressive lens is carefully optimized during the lens design process in order to minimize astigmatism, image swim, skew distortion, and other unwanted features. A well-designed progressive lens will reduce unwanted astigmatism and its associated effects to its mathematical limits. Generally, the maximum level of unwanted astigmatism in a modern progressive lens will be similar in magnitude to the Add Power, so that a +2.00 Add progressive lens will frequently have a maximum level of astigmatism of roughly 2.00 D. Image swim, which can result in the apparent acceleration of images, can be minimized by controlling the rates of change in prism and surface power, particularly along meridians that are orthogonal (perpendicular) to the line of sight. Skew distortion can be minimized by controlling the axis of the unwanted astigmatism in the periphery so that it is generally oriented at a less oblique axis. Lens Design SymmetryEarly progressive lenses were symmetrical in design, so that the right and left lenses were identical. To achieve the desired inset for the near zone, the lens blanks were simply rotated 9 to 11°. The principal drawback to this approach was the disruption of binocular vision as the wearer gazed laterally across the lens, since the astigmatism and power differed between the nasal and temporal sides of the distance zone. The binocular field of view, or the combined region of clear vision seen simultaneously through both lenses, was also severely restricted. 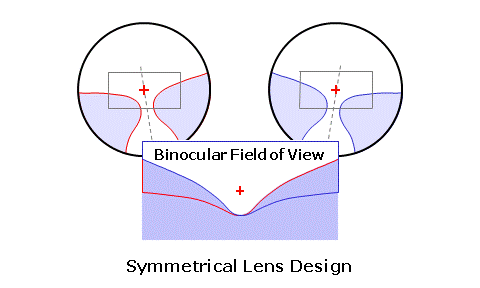 Most modern lens designs, however, are now asymmetrical in design, with separate right and left lens designs. The amount of astigmatic error on either side of the progressive corridor can now be adjusted independently. This allows the near inset to be achieved without rotating the lens design; instead, the umbilic is designed from the start with a slight nasalward inclination. This reduces the disruption in binocular vision, and provides better overlap between the distance, intermediate, and near viewing zones of the right and left lenses—affording a larger binocular field of view. 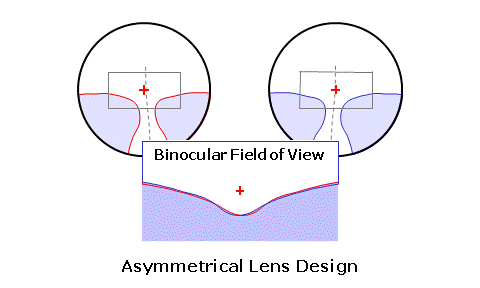 While asymmetrical designs improve binocularity and ensure a better binocular field of view, levels of power and unwanted astigmatism may be higher to the nasal side of the progressive corridor as a result of achieving the near inset without rotating the design. Consequently, unless this unwanted power and astigmatism are carefully managed, differences in prism, magnification, and power may still exist between corresponding points on the two lenses lenses as the eyes move across them. Since most vision tasks involve both eyes working together in unison, these binocular differences may result in less comfortable binocular fusion. Horizontal symmetry extends the notion of an asymmetrical lens design by minimizing the differences in power between points at equal lateral distances to the right and left of the eyepath, essentially "mirroring" the optics of the surface horizontally at each point along the umbilic. This improves binocular performance by reducing the differences in power between the right and left lenses for corresponding points. Further, by minimizing power differences between corresponding points on the lenses, the two eyes perceive virtually no difference in prism, blur, and magnification, which ensures more comfortable binocular vision. 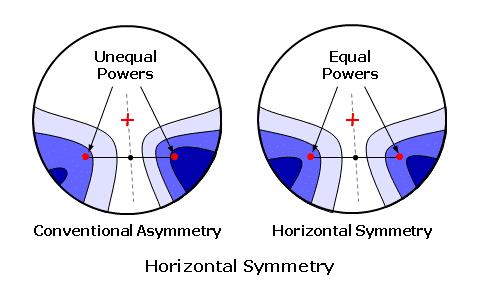 Multi-Design LensesOriginally, progressive lenses employed a single unique lens design that was essentially scaled for each Base curve and Add power combination in its range of semi-finished lens blanks. The lens design used for the steepest Base curve was identical to the lens design used for the flattest Base curve; the progressive surface was simply "scaled" up. Similarly, the lens design used for the highest Add power was simply scaled up in a linear fashion from the design used for the lowest Add power. For instance, the lens design for the +2.00 Add power had twice as much surface astigmatism as the +1.00 Add. A progressive lens product that utilizes the same basic lens design across every Base curve and Add power combination is now referred to as a mono-design lens series. 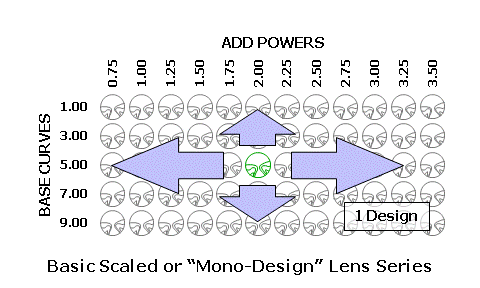 While a mono-design lens series represents the easiest approach to progressive lens design, lens designers soon realized that the overall performance of the lens series could be improved by tailoring the lens design slightly in order to account for optical effects that were specific to the wearer's distance prescription and/or stage of presbyopia. For instance, the lens design could be adjusted by Base curve to compensate for the differences in magnification or prism produced between different distance prescriptions. Similarly, the design could be adjusted by Add power to compensate for the differences in surface astigmatism produced between different Add powers. The first stage in the evolution from traditional mono-design lenses occurred in the late 1980s, when lens designers started adjusting the basic progressive lens design for each Add power. A progressive lens product that utilizes a different basic lens design for each Add power is now referred to as a multi-design lens series. The first multi-design lenses employed softer designs in the low Add powers in order to provide emerging presbyopes with a more single vision-like visual experience, while employing harder designs in the higher Adds in order to offer greater viewing zone utility for advanced presbyopes. 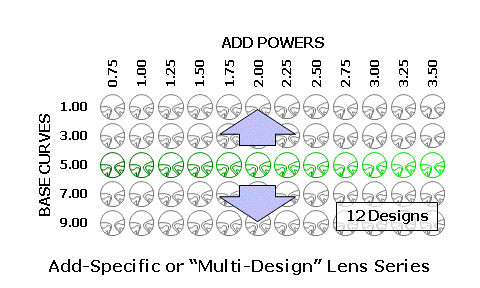 The next stage in the evolution of progressive lenses occurred in the mid 1990s, when lens designers began adjusting the basic progressive lens design by both Base curve and Add power. A progressive lens product that utilizes a different basic lens design for each Base curve and Add power combination is often referred to as a Design by Prescription lens series. In addition to changes based on Add power, the earliest Design by Prescription lenses employed designs with slightly larger near zones in the steeper Base curves to compensate for the increased magnification and reduced field of view produced by plus prescriptions. This also allowed the flatter Base curves to have slightly larger distance zones, which reduced the loss in distance vision that results from the interaction of the progressive surface astigmatism with the oblique aberrations produced by minus prescriptions.  A mono-design lens series uses a single basic design that is simply scaled for each Base curve and Add power combination. For a range of Add powers from +0.75 to 3.50 D, a typical multi-design lens series could result in up to 12 unique lens designs. For 5 Base curves and a range of Add powers from +0.75 to 3.50 D, a typical Design by Prescription lens series could result in up to 60 unique lens designs. Variable Near InsetConventional progressive lens designs employ a fixed, 2.5-mm inset for each near zone. This approach has several limitations, and does not take into consideration how the wearer's reading distance or prism from the distance prescription will affect ocular convergence:
 These factors will influence the interpupillary distance required during near vision, which means that the inset of the progressive near zone should be adjusted accordingly. Many modern progressives now use a variable inset that adjusts the inset of the near zone for each Base curve and Add power combination. Using this approach, the exact near inset required for each base curve and add power are carefully computed to ensure that the centers of the near zones are properly aligned with the lines of sight during near vision. This improves the wearer's binocular field of view through the near zone. Typically, flatter Base curve—which are used for minus powers—are designed with smaller near insets. Conversely, steeper Base curves will have greater near insets. Since the reading distance of the bifocal segment or near zone can be no longer than the reciprocal of the Add power, higher Add powers require shorter working distances. Consequently, higher Add powers are typically designed with greater near insets. A low-Add, low-Base lens may only require a near inset of 1½ mm, while a high-Add, high-Base lens may require 3½ mm or more of near inset. As-Worn OptimizationMany progressive lenses utilize flatter base (or front) curves to provide flatter, thinner, and lighter lenses. In order to maintain accurate peripheral optics by minimizing the aberrations introduced by using flatter lens forms, the distance zone of the lens may be aspherized. Aspheric surfaces use surface astigmatism to neutralize the oblique astigmatism introduced by viewing through the periphery of the lens. The application of asphericity to the distance viewing zone of a progressive lens allows the use of flatter, thinner lenses, without unnecessarily compromising the peripheral optical performance of the lens design. Another recent innovation in progressive lens design is the process of optically optimizing the lens design for the as-worn position or position of wear, which represents how the lenses are actually worn. This process uses optical ray tracing and lens-eye modeling to refine the optical powers as perceived by the wearer with the lenses in their intended positions. This takes into account the influence that oblique aberrations, lens tilt, vertex distance, and other variables affecting viewing conditions have on the optical powers perceived by the wearer. For instance, tilting a high-powered lens effectively increases the sphere power and introduces cylinder power.  Conventional progressive lenses, on the other hand, are designed to maintain distance and near zones that are optically clear when measured for vertex power by standard focimeters—including vertometers and lensometers—not as seen by the actual wearers. Consequently, as-worn optimization ensures that the lenses will deliver the prescribed powers during actual use. In some cases, these lenses may arrive with a compensated prescription, which is the prescription you should verify in a focimeter. A lens that produces the correct compensated Rx in a focimeter will produce the correct prescribed Rx for the wearer once the lenses are in their "as-worn" positions. A single Base (front) curve can only deliver optimal peripheral optical performance for a single prescription power. Generally, this single power is a sphere power located toward the middle of the prescription range recommended for that particular Base curve. As the wearer's prescription deviates from this single "optimal" power, the optical performance of the lens design deteriorates. When cylinder power has been prescribed, this deterioration in optical performance can also result in an effective shift of the central viewing zones. Fortunately, free-form surfacing surfacing frees lens designers from the constraints of traditional Base curve limitations. Free-form surfacing equipment can generate both simple and complex surfaces, including progressive lens designs, directly onto a lens blank "on-the-fly." When used in combination with sufficiently advanced optical design software, free-form surfacing technology allows a unique lens design to be created for each wearer. Certain free-form progressive lenses are individually optimized for the wearer's specific combination of prescription and fitting (position of wear) parameters. This ensures that every pair of lenses performs exactly as intended. Prism-ThinningEarlier, it was demonstrated that the curvature of a progressive lens surface must become increasingly steeper towards the bottom of the lens in order to provide a gradual—or progressive—change in power. This surface geometry has several important consequences for a progressive lens blank:
Fortunately, it is possible to minimize the excess thickness and weight of progressive lenses. A technique known as prism-thinning can be employed to improve the cosmetics of a progressive lens. Prism-thinning (also called equi-thinning) is the process of grinding prism into a progressive lens blank in order to minimize the thickness difference between the top and bottom edges of the lens, as well as to reduce the overall thickness of the lens. 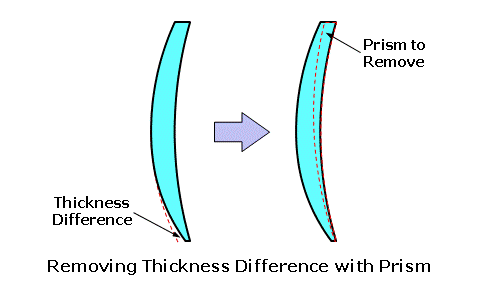 Most commonly, prism-thinning typically involves grinding base down prism into a progressive lens in order to remove a wedge of base up prism from the lens blank. Prism-thinning is particularly beneficial for plus lenses and lenses with high Add power, but may also be applied to minus lenses when necessary. Prism-thinning should be treated like prescribed prism, and verified at the prism reference point of each lens. |

