Ray Tracing of Thin Lenses

By Darryl Meister

Introduction

This course will present the fundamentals of simple ray tracing of "thin" optical lenses, including a review of conjugate points, focal distance, and linear magnification. This is a technical, advanced level course intended for dispensing opticians, laboratory technicians, and paraoptometric personnel. An understanding of both basic algebra and intermediate optical theory is required.

The Thin Lens

Ray tracing is the process of graphically determining the size and position of an image after refraction through a lens, and is useful for developing a solid intuition about the optics of lenses, as well as for verifying actual mathematical calculations. This course will demonstrate the ray tracing procedures for a thin lens, which is a lens whose center thickness and curvature are negligible—allowing us to ignore them in our calculations. This will simplify our work tremendously. For our purposes, we will make the following assumptions for this course:

• That the lens is infinitely thin, which allows us to ignore the effects of lens thickness on power
• That the lens is surrounded by air, which has a refractive index of approximately 1
• That the object rays are paraxial, or near the optical axis of the lens, which allows us to ignore aberrations

Furthermore, ray tracing diagrams, and the derivation of optical formulas in general, generally follow a strict optics sign convention in order to ensure consistent calculations. If you are unfamiliar with this sign convention, you can review it in the Tech Topics, below.

Image Formation Review

One of the principal concepts in geometrical optics is the idea of conjugate pairs. Essentially, every object point produces a corresponding—or conjugate—image point after refraction through the lens. The term conjugate points simply refers to these corresponding object and image point pairs. An object at point (L) produces an image at its conjugate image point (L') after refraction through the lens. The initial vergence of light (L) from the object point at the plane of the lens is equal to the reciprocal of the object distance (l) in meters:

L = 1 / l and l = 1 / L

The final vergence of the light (L') approaching the image point at the plane of the lens is equal to the reciprocal of the image distance (l') in meters:

L' = 1 / l' and l' = 1 / L'

The image position (L') of an image after refraction through the lens is given by the conjugate foci formula:

L' = L + F

This formula simply states that the final image vergence (L') leaving a lens is equal to the net effect of the focal power (F) of the lens on the initial object vergence (L) entering the lens.

For example, consider an object 50 cm (0.5 m) to the left (negative vergence) of a +4.00 D lens. The object vergence L is equal to 1 / l = 1 / -0.5 = -2.00 D. The final image vergence L' is equal to F + L = -2.00 D + 4.00 D = +2.00 D. Consequently, the image distance l' from the lens is equal to 1 / L' = 1 / +2.00 = +0.5 m (a real image located 50 cm to the right of the lens).

Lateral Image Magnification

The lateral magnification (m) of an image is simply the ratio of the image size (h') to the object size (h):

m = h' / h

Using similar triangles, we can show that the lateral magnification (m) for conjugate object and image distances (l and l') is also given by:

m = l' / l

And, after substituting the object and image vergence equivalents (L and L'):

m = L / L'

Lateral magnification is simply a ratio that compares the relative size of the final image to its intial object. It can be expressed as a factor (e.g., 2x) or as a percentage (e.g., 200%). When the magnitude of the magnification (m) is equal to 1, which is unity, the object and image are the same size. When the magnitude is greater than 1, the final image is larger than the initial object, or magnified. Conversely, when the the magnitude is less than 1, the image is smaller than the object, or minified.

Note that, since the lateral magnification m = l' / l, the image is larger than the object when the image distance is longer than the object distance. Conversely, the image is smaller than the object when the image distance is shorter than the object distance. Moreover, the following rules apply to the sign of the final magnification value:

• Positive (+) values for the magnification (m) represent images that are erect, or oriented the same way, relative to their initial objects.
• Negative (-) values represent images that are inverted, or rotated 180°, relative to their initial objects.

Image Calculations

The fields, below, will automatically perform some simple image calculations for conjugate points. Simply enter in the object distance in meters and the focal power of the lens in diopters, and then press the Calculate button. The object vergence at the plane of the lens in diopters, the image vergence in diopters, the image distance in meters, and the lateral magnification will all be displayed.

Object Distance (m)?
Focal Power (D)?
Object Vergence (D):
Image Vergence (D):
Image Distance (m):
Lateral Magnification (x):

Note that, according to our optical sign convention, real objects—which are to the left of the lens in our ray tracing diagrams—should have a negative (-) object distance.

Three Construction Rays

Once you understand some of the fundamental principles of lenses, ray tracing becomes quite straightforward. We first assume that the object lies on the optical axis of the lens. Since the bottom point of the object lies on the optical axis, it passes through the lens without deviation and defines the vertical location of the bottom point of the image.

Next, we judiciously select three specific rays that we will trace from the top point of the object and through the lens. We will choose three rays whose paths we can actually predict after refraction through the lens. Where these three rays intersect denotes the horizontal and vertical location of the top point of the image, allowing us to completely define the size and position of the final image.

If the object is located at the primary focal point (F) of the lens, the rays will not intersect but remain parallel instead. This will create an image at optical infinity. The selection of these rays is based upon three principles that we will examine, each of which produces its own conjugate set of construction rays:

1. Primary focal point: An object positioned at the primary focal point (F) of a lens will produce an image at infinity. This means that rays of light originating at the primary focal point (F) are rendered parallel after refraction through the lens. Consequently, any ray of light passing through the primary focal point is rendered parallel to the optical axis after refraction through the lens. The primary focal length (f) in meters, which is the distance between the primary focal point and the lens, is given by f = 1 / F. This is our first unique pair of construction rays.
2. Secondary focal point: An object positioned at optical infinity will produce either a real image (for a plus lens) or a virtual image (for a minus lens) at the secondary focal point (F'). This means that parallel rays of light are brought to a focus at or appear to originate from the secondary focal point (F') after refraction through the lens. Consequently, any ray of light parallel to the optical axis will pass through the secondary focal point after refraction through the lens. The secondary focal length (f') in meters, which is the distance between the secondary focal point and the lens, is given by f' = -1 / F'. This is our second unique pair of construction rays.
3. Optical center: Any ray of light passing through the optical center (O) of a lens will leave the lens parallel to its original path. The optical center is the point on the lens free from any prism. For thin lenses, any ray of light passing through the optical center will be completely undeviated after refraction through the lens. This is our last unique pair of construction rays, although—since the ray passes through the lens undeviated—it is actually a single ray, not a pair. This ray is also referred to as the chief ray.

Note that, for a thin lens surrounded by air, the primary focal length (f) is equal in magnitude to the secondary focal length (f'), so that f = -f'. This means that these two points will be equidistant from, but on opposite sides of the lens. Also note that the order of these construction rays is not important. We will now demonstrate how these three construction ray sets are used for ray tracing objects through several lenses.

Ray Tracing a Plus Lens

In our first example, a real object is located at a point (L) beyond the primary focal point (F) of a plus lens, so that the object distance is greater than the primary focal length (l > f). Note that the bottom of the object is positioned along the optical axis for convenience. This delimits the bottom of the image.

Primary focal point: We begin by tracing a ray from the tip of the object through the primary focal point (F) of the lens and to the plane of the lens itself. This ray will be rendered parallel to the optical axis after refraction through the lens.

Secondary focal point: Next, we trace a ray from the tip of the object and parallel with the optical axis to the plane of the lens. This ray will be deflected through the secondary focal point (F') after refraction through the lens.

Optical center: Finally, we trace a ray from the tip of the object and through the optical center (O) of the lens. This ray will remain straight and undeviated after refraction through the lens.

All three rays should now be intersecting each other—unless the image is at infinity. The point of intersection represents the position of the top point of the image, which may be inverted (or rotated upside down) relative to the original object. The image can now be drawn from the optical axis of the lens to this point of intersection.

If this ray trace was done to scale, the object and image sizes (h and h') and distances (l and l') can be measured directly. Note that the image (h') lies below the optical axis, which indicates a negative (-) value. In this case, a real image is formed, which is inverted relative to the original object. This means that the image can be focused upon a screen, and will be rotated 180°.

Also note that the image height (h') is smaller than the object height (h). This image size and orientation would also be confirmed with the lateral magnification formula, which would produce a negative value with a magnitude of less than 1. In general, real objects located beyond the secondary focal point of a plus lens will produce real images that are inverted and either magnified or minified, depending upon the object distance.

Now consider an example in which the object is located at a point (L) within the primary focal point (F) of a plus lens, so that the object distance is less than the primary focal length (l < f). The three constructions ray sets are drawn again, but with some slight variations.

In this case, the primary focal point construction ray is drawn from the primary focal point F first, and then through the tip of the object to the plane of the lens. Moreover, the three construction rays must then be projected backwards from their refracted paths to find their common point of intersection.

Note that the three construction rays still intersect to form a single image point that represents the top point of the image. In this case, a virtual image is formed, which is erect relative to the original object. Also note that the image height h' is larger than the object height h. In general, real objects located within the secondary focal point of a plus lens will produce virtual images that are magnified. This is the principle behind hand magnifiers and stand magnifiers.

Ray Tracing a Minus Lens

Ray tracing a minus lens is similar to ray tracing a plus lens with a virtual image, since the construction rays must also be projected backwards from their refracted paths to find their common point of intersection. There is one significant difference between ray tracing plus and minus lenses; the primary and secondary focal points (F and F') are on opposite sides of the lens. For plus lenses the primary focal point F lies in front of the lens (to the left), while the primary focal point F lies behind the lens (to the right) for minus lenses. In this example, a real object is located at a point L beyond the secondary focal point F' of a minus lens, so that the object distance is longer than the secondary focal length.

Primary focal point: We begin by tracing a ray from the tip of the object and towards the primary focal point (F) on the opposite side of the lens. This ray will be rendered parallel to the optical axis after refraction through the lens. We will need to extend this parallel ray backwards, since the virtual image will lie in the object space.

Secondary focal point: Next, we trace a ray from the tip of the object and parallel with the optical axis to the plane of the lens. This ray will be deflected so as if to have originiated at the secondary focal point (F') after refraction through the lens. Again, we will need to project the refracted ray backwards into object space.

Optical center: Finally, we trace a ray from the tip of the object and through the optical center (O) of the lens. This ray will remain straight and undeviated after refraction through the lens.

All three rays should now be intersecting each other—unless the image is at infinity. The point of intersection represents the position of the top point of the image. The image can now be drawn from the optical axis of the lens to this point of intersection.

In this case, a virtual image is formed, which is erect relative to the original object. This image cannot be focused upon a screen, but can serve as an object for another lens or system (such as the eye). Also note that the image height (h') is smaller than the object height (h). In general, real objects refracted by a minus lens will produce virtual images that are minified.

Next, we will examine the process of ray tracing a virtual object through a minus lens. Recall that virtual objects are formed in image space. Instead of diverging from a real object point, rays of light converge to form a virtual object point, which is located in image space. Ray tracing virtual objects can be a bit more challenging at first, since you are not drawing rays directly from the object. Instead, you are drawing rays toward the intended location of the object, which will be intercepted by the lens. In this example, a virtual object is located at a point (L) behind a minus lens (to the right) and within the primary focal point (F), so that the object distance is shorter than the primary focal length (l < f).

The three construction ray sets are again drawn, but the process may seem somewhat reversed. Each construction ray must be initially directed towards the tip of the object, before being intercepted by the lens. Moreover, the three construction ray sets must still be chosen to pass or extend through the primary focal point, secondary focal point, and optical center after refraction through the lens.

In this case, a real image is formed, which is erect relative to the original object. Also note that the image height (h') is larger than the object height (h). In general, virtual objects located within the primary focal length of a minus lens will produce real images that are magnified. Virtual objects located beyond the primary focal point of a minus lens will produce virtual images that are inverted and either minified or magnified, depending upon the object distance.

Ray Tracing an Object at Infinity

So far, we have examined situations in which the object is at some finite point from the lens. However, if the object is located at infinity, the object distance (l) is also infinite. Consequently, you can no longer solve the lateral magnification formula (m = l' / l) or ray trace a lens using the methods described so far. However, it is possible to calculate the image size (h'), as well as to ray trace the object through the lens, if we know the angle that the object subtends from the optical axis of the lens. Recall that, for an infinitely distant object, the image is located at the secondary focal point (F') of the lens, so that l' = f'. If the object subtends the angle (s) with the optical axis of the lens, we can use the primary focal point and optical center construction rays to ray trace the object.

We begin by tracing a ray through the optical center (O) of the lens that forms the angle (s) with the optical axis. Then we trace another ray, parallel to the first and forming the same angle (s), through the primary focal point (F) and to the lens, where it will be rendered parallel to the optical axis after refraction. These two rays will intersect in the plane of the secondary focal point (F') to form the top point of the image.

From the diagram, we can see that the image formed at the secondary focal point (F') has a height (h') given by:

h' = -f' × tan s

And, after substituting 1 / F for f',

h' = -tan s / F

For example, consider an object at infinity that subtends a 30° angle from the optical axis of a +3.00 D lens. The height (h) of this image will be equal to -tan s / F = -tan 30° / +3.00 = -0.19 m. (Recall that the negative value for the image size indicates that the image will be inverted relative to the object.)

Plus Lens Ray Tracing Experiment

One way to develop a more solid intuition regarding the relationship between objects and their corresponding images is to observe the relationship between a range of object points and their conjugate image points. We will use a plus lens for this example, since many practical uses of ray tracing—including the evaluation of the magnification produced by low vision aids—often involve the analysis of plus lenses.

Each arrow below represents an object position between infinity and the lens. Click on different object positions to see the resulting image distance, size, and orientation.

The first position represents an object at infinity, producing a real, inverted image at the secondary focal point (F'). The second position represents an object within infinity but beyond the primary focal point (F), producing a real, inverted image beyond the secondary focal point (F'). The third position represents an object at the primary focal point (F), producing an image at infinity. The fourth position represents an object within the primary focal point (F), producing a virtual, erect image.

Ray Tracing a Thin Prism

We will complete our ray tracing excercises by tracing an object ray through a thin prism, which is generally a prism with an apical angle of less than 15°. For simplicity, we will assume that the initial object ray is striking the first surface normally (or perpendicularly). Since the ray is normal—or at a 90° angle—to the first surface, no refraction occurs. However, once the ray strikes the second surface, it reaches the surface at an angle i and is therefore refracted (or bent) in the direction of the base.

For thin prisms, which include most prisms in ophthalmic optics, the refraction at the second surface depends primarily on the apical angle (a) of the prism and the refractive index (n) of the material. In practice, the angle of incidence at the first surface will affect the extent to which light is deviated by the prism. The amount of deviation, in degrees, is given by the angle (d), while d = i' - i.

Moreover, the angles of incidence (i) and refraction (i') are related by the refractive index (n) of the prism material according to Snell's law. For a thin prism, with a relatively small apical angle (a), it can be shown that the approximate deviation (d), in degrees, is given by:

d = (n - 1) × a

For small amounts of deviation, 1 degree of deviation is roughly equal to 1.75 prism diopters. For prisms made from hard resin, the refractive index (n) is 1.500. This simplifies our formula even further, since 1.500 - 1 = 1/2. Consequently, for prisms made from hard resin, the deviation in degrees is roughly equal to half the apical angle.

For example, consider a ray of light from an object point passing through a hard resin prism (n = 1.500) with an apical angle of 10°. The deviation (d) of this ray is equal to (n - 1) × a = (1.500 - 1) × 10 = 5°. This is approximately 8.75 prism diopters.

Sketching Ray Traces

Now that you have been shown the fundamentals of ray tracing, you can begin sketching your own diagrams. A sheet of graph paper works nicely for this purpose. The horizontal and vertical distances should be drawn to scale, though they may use different scales. Ensure that your horizontal scale allows you to fit the entire diagram on the page, including object and image distances. (The vertical axis isn't as critical since the relative object/image size can still be measured.) For simplicity, a double-ended line with arrowheads is used to represent the lens; arrowheads facing opposite directions are used for plus lenses, while arrowheads facing toward each other are used for minus lenses.

Begin by drawing an optical axis, and then place the lens, optical center, focal points, and object at their appropriate locations along the axis. Care should be taken to place the focal points and conjugate points accurately along the optical axis. (The primary and secondary focal lengths should be calculated.) Next, draw an arrow that represents the object, and begin ray tracing your three construction ray sets.

Although this course has demonstrated the principles of ray tracing a single thin lens, the techniques described here are also applicable to systems with multiple lenses. To ray trace objects through optical systems with multiple lenses, simply ray trace each lens individually to determine its final size and position. Each ray-traced image becomes the new object for each subsequent lens. The process is repeated until the final object and image pair is ray traced for the last lens in the system, which will provide the final image size and position for the entire system.

This can also be done solely on the basis of simple calculations, by calculating the image vergence and lateral magnfication at each lens. (Again, each new image becomes the object for the next lens in the system.) The total lateral magnification of the system is simply product of all the individual lateral magnification values at each lens.

Appendix: Optical Sign Convention

Ray tracing diagrams, and the derivation of optical formulas in general, generally follow a strict optics sign convention in order to ensure consistent calculations:

• Light travels from left to right.
• The region to the left side of the lens is referred to as the object space, and real objects are positioned on this side.
• The region to the right side of the lens is referred to as the image space, and real images are located on this side.
• An image located in object space is referred to as a virtual image (this situation often occurs with minus lenses).
• An object located in image space is referred to as a virtual object (this situation only occurs when something affects the vergence of light before reaching the lens).
• The lens serves as the center (0) of the coordinate system, which can be thought of as a simple number line (i.e., negative numbers on the left, positive on the right).
• Distances measured from the left side of the lens into object space are considered negative (-), while distances measured from the right side into image space are positive (+).

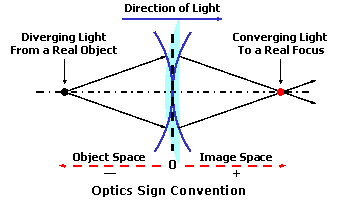Note that light diverges (negative vergence) from a real object point in object space, and converges (positive vergence) to a real image focal point in image space. If light continues to diverge in image space, a virtual image point is produced in object space.